Tên đề tài luận án tiến sĩ: Nghiên cứu thuật toán giảm bậc mô hình và ứng dụng cho bài toán điều khiển

Chuyên ngành: Kỹ thuật Điều khiển và Tự động hóa

Mã số: 62.52.02.16

Khóa đào tạo: 2011 - 2015

Họ và tên NCS: Vũ Ngọc Kiên

Họ và tên người hướng dẫn khoa học:

            1: PGS. TS. Nguyễn Hữu Công;

            2: PGS. TS. Bùi Trung Thành.

Đơn vị đào tạo: Trường Đại học Kỹ thuật Công nghiệp

Cơ sở đào tạo: Đại học Thái Nguyên

NHỮNG KẾT QUẢ MỚI CỦA LUẬN ÁN

Luận án đã có những đóng góp mới sau:

1. Đưa ra 3 tiêu chuẩn đánh giá (đo) tính quan trọng (tính trội) của các điểm cực và xây dựng thuật toán giảm bậc mới cho hệ ổn định sử dụng 3 tiêu chuẩn này để đánh giá và sắp xếp các điểm cực theo tính quan trọng (tính trội) giảm dần trên đường chéo chính của ma trận tam giác trên , bằng cách này, có thể bảo toàn được các điểm cực quan trọng của hệ gốc trong hệ giảm bậc đồng thời thu được sai số giảm bậc nhỏ. Thuật toán mới này cũng được mở rộng áp dụng giảm bậc cho hệ không ổn định theo phương pháp gián tiếp. Song song với đó, luận án cũng đưa ra được một định lý mới xác định công thức tính chặn trên của sai số giảm bậc, 5 bổ đề mới và các ví dụ minh họa để minh chứng tính đúng đắn và hiệu quả của thuật toán mới.

2. Xác định công thức tính chặn trên của sai số giảm bậc cho thuật toán chặt cân bằng mở rộng để giảm bậc hệ không ổn định giúp việc đánh giá sai số giảm bậc dễ dàng hơn và thực hiện giảm bậc tự động dựa vào công thức tính chặn trên của sai số giảm bậc. Đồng thời, luận án cũng đưa ra được 2 định lý mới và một ví dụ giảm bậc mô hình tuyến tính không ổn định để chứng minh tính đúng đắn và hiệu quả của thuật toán.

CÁC ỨNG DỤNG, KHẢ NĂNG ỨNG DỤNG TRONG THỰC TIỄN VÀ NHỮNG VẤN ĐỀ CẦN TIẾP TỤC NGHIÊN CỨU

* Các ứng dụng và khả năng ứng dụng thực tiễn:

- Ứng dụng hai thuật toán giảm bậc trong việc giảm bậc bộ điều khiển bậc cao cho kết quả:

+ Với bài toán thứ nhất là giảm bậc bộ điều khiển bền vững bậc cao trong bài toán điều khiển bền vững góc tải của máy phát đồng bộ trong hệ thống điện: Sử dụng bộ điều khiển bậc 4 thay thế bộ điều khiển gốc bậc 28 mà chất lượng hệ thống điều khiển vẫn được đảm bảo, các kết quả đã được kiểm chứng qua mô phỏng trên máy tính.

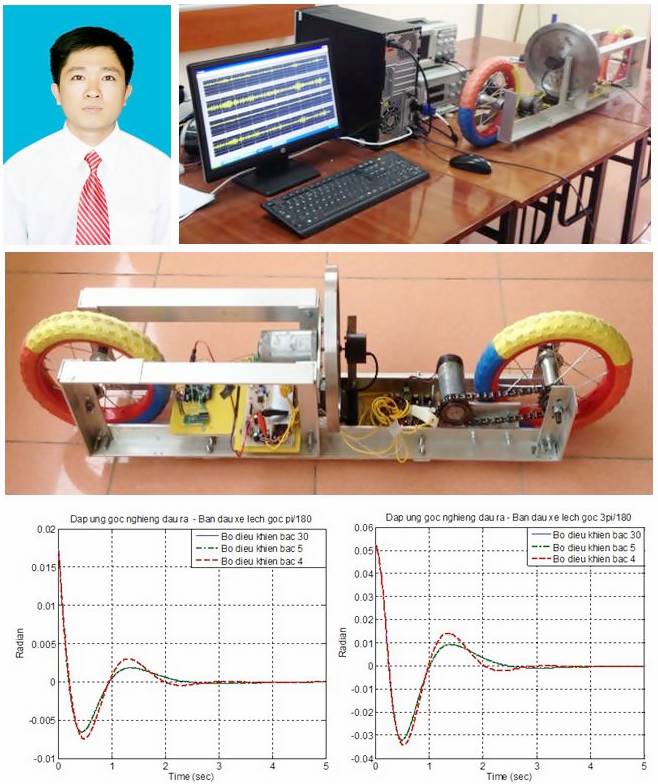
+ Với bài toán thứ hai là giảm bậc bộ điều khiển bền vững bậc cao trong bài toán điều khiển xe hai bánh tự cân bằng: Sử dụng bộ điều khiển bậc 5, bậc 4 thay thế bộ điều khiển bền vững bậc 30 mà chất lượng hệ thống điều khiển vẫn đảm bảo được yêu cầu ổn định bền vững của hệ thống. Các kết quả đã được kiểm chứng qua mô phỏng trên máy tính và thực nghiệm trên mô hình xe hai bánh.

- Kết quả nghiên cứu giúp đơn giản hóa các mô hình bộ điều khiển bậc cao hoặc mô hình đối tượng bậc cao từ đó sẽ giảm khối lượng tính toán cần được xử lý, giảm dung lượng lưu trữ dữ liệu và dung lượng bộ nhớ của phần cứng trong mô phỏng và điều khiển, giảm chi phí kinh tế hoặc khai thác hiệu quả các hệ thống cũ, các hệ thống có kết cấu nhỏ gọn và cấu hình phần cứng thấp.

 - Kết quả nghiên cứu là tài liệu tham khảo hữu ích cho đào tạo và nghiên cứu của cán bộ, giảng viên, sinh viên khi nghiên cứu về giảm bậc mô hình và thiết kế bộ điều khiển bền vững bậc thấp.

 * Vấn đề bỏ ngỏ cần tiếp tục nghiên cứu:

- Nghiên cứu ứng dụng hai thuật toán giảm bậc mô hình mới để giải quyết các bài toán giảm bậc mô hình khác trong lĩnh vực điều khiển như giảm bậc mô hình đối tượng bậc cao.

- Xây dựng toolbox của hai thuật toán giảm bậc trong Matllab – Simulink.

INFORMATION OF DOCTORAL DISSERTATION

Dissertation title: Researching model order reduction algorithm and applying to control problem

Speciality:  Control Engineering and Automation

Code: 62.52.02.16

Ph.D candidate: Vu Ngoc Kien

Training course: 2011 - 2015

Scientific supervisors:      Assoc. Prof. Nguyen Huu Cong, PhD.

                                                Assoc. Prof. Bui Trung Thanh, PhD.

Training institution: College of Technology - Thai Nguyen University

THE NEW SCIENTIFIC FINDINGS

            The dissertation has the following new contributions:

1. Giving three evaluation criterias (measuring) the importance (dominance) of the poles and building new algorithms for stable system applied these three evaluation criterias to evaluate and arrange the poles follow the descending important (dominant) properties on the main diagonal of the upper – triangle matrix , by this way,the dominant poles of original system in order reduction system can be preserved, also obtaining small error reduction as well.. This algorithm is also applied for unstable system follow indirect method.Along with that, the dissertation also provided a new theorem whichdetermined the upper bound formula of order reduction error, 5 new lemmas and illustrative examples to prove the correctness and the effectiveness of the new algorithm.

2. Determining the upper bound formula of order reduction error for extend balance truncation algorithm to reduce order of unstable system that helps to easier evaluate order reduction error and  perform automatic order reduction based on the upper bound formula of order reduction error.Besides, the dissertation also provided 2 new theorems and an example to prove the correctness and the effectiveness of the new algorithm.

APPLICATIONS, PRACTICAL APPLICABILITY AND THE NEEDS FOR FURTHER STUDIES

* Applications and applicability in practice:

- Applying two algorithms in reducing high order controller showed results as below

+ For the first problem which is reduced order of high-order robust controller in robust load angle control problem of synchronous generators:  applying the 4^th-order controller to replace the original 28^th-order one but the quality of controller is still guaranteed. Theresults have been verified through computer simulations.

+ For the second problem which is reduced order of high-order robust controller in balance control  of two-wheeled bicycle problem: Applying the 5^th,4^th-order controller to replace the 30^th-order one but the quality of controller is still guaranteed. The results have been verified through computer simulations and experimented on two-wheeled bicycle model.

- The results of research help to simplify higher-order controller or higher-order object model so that reduce the volume of calculations need to be processed, the data storage capacity and memory capacity of the hardware in simulation and control, decrease economic cost or effectively running the old system; the small structured systems with lower hardware configuration.

- The result of the research is useful reference for training and reseach of officers, lecturers, students when studying model order reduction and lower-order robust control design.

* Issues needing for further studies:

1. Study to apply two new algorithms to solve model order reduction problems in  control field such as reduce high-order object model.

 2. Building toolbox of two new algorithms in Matllab – Simulink.